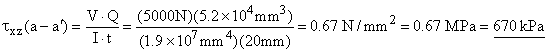Example 6.4.1

Given: The rectangular beam, built in at the left end, having length, L, and cross-section of width, b, height, h, is acted upon by a point load, P, at its free end.

Req'd: Determine the shear stress at the top, bottom and neutral axis at a cross-section in the beam.

Sol'n: The shear force, V(x) = P, is constant across the entire beam. At the top (or bottom) surface (y = ±h/2), y^* = h/2 and A^* = 0, therefore t = 0.

At the neutral axis (y₁ = 0), y^* = h/4 and A^* = bh/2. Thus:

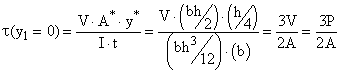
The shear distribution, calculated as a function of y (=y₁), is a parabola, given by the function:

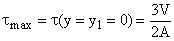
Note that these equations for t(y) are only valid for beams of rectangular cross-section.

Note: shear-stress acts parallel to the beam-face. The parabola is a plot of the magnitude of the shear stress.

Example 6.4.2

Given: The I-beam at right is subjected to shear force, V = 5 kN. The flange and the web both have a thickness of 20 mm, the height of the beam is 150 mm and the width is 100 mm. The beam has a moment of inertia of I = 1.9x10⁷ mm⁴.

Req'd: Determine the shear stress at a-a' and b-b'.

Sol'n: The first moment of area (about the neutral axis of the entire cross-section) of the area to the left of a-a' is:

Q = A^*·y^* = (40 mm · 20 mm)·65 mm = 5.2x10⁴ mm³

y* =[ (150/2 mm) - (20/2 mm)] = (half the height of the cross section) minus (half the thickness of the flange).

Then the shear stress is in the flange is:

The first moment of area of the area to the below of b-b' is:

Q = A^*·y^* = (100 mm· 20 mm) (65 mm) = 1.3x10⁵mm³

And the shear stress in the web just above (below) the flange, is: