


| Practice Problem 2-5 |



|
|
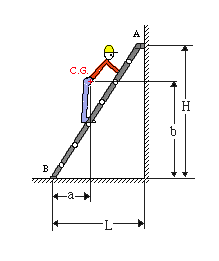
Given: A painter with weight, Wman = 180 lb, stands on a ladder. The ladder has a weight of Wladder = 40 lb. The center of gravity (C.G.) of the painter is located at a = 2.5 ft and b = 6 ft. Let L = 6 ft and H =12 ft. Req'd: |
|
|
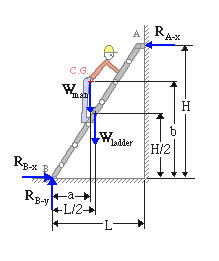
Solution: First create a FBD of the system. Since Point A acts as a roller, the only reaction force is in the x-direction, RA-x. Point B has reaction forces in both the x- and y-directions, RB-x and RB-y. Note that the weight of the man, Wman, acts at the C.G. of the man while that of the ladder acts at the mid-point (C.G.) of the ladder, L/2 and H/2. Next apply equilibrium equations to determine the reaction forces: SFx = 0: RA-x = RB-x SFy = 0: RB-y = Wman + Wladder SMB = 0: RA-x = [(Wman)(a) + (Wladder)(L/2)] / H = 47.5 lb Therefore, RB = (RB-x2 + RB-y2)1/2 = 225 lb |
|

|



|